ESTRO 36 Abstract Book

232 / 1082

S219 ESTRO 36 2017
_______________________________________________________________________________________________
calibration factors for the chamber at other beam
qualities whereby correction factors for a particular beam
quality are determined by interpolation. When no
experimental kQQo values are provided, the user can
calculate them by using a set of expressions derived from
Bragg-Gray theory or obtain k QQo values by Monte Carlo
(MC) simulation. Analytical and MC kQQo values are derived
from the nominal geometry of each chamber model since
there is no way of knowing the exact dimensions of each
user chamber. In contrast, calibration in terms of
absorbed dose to water in a SDL, at different beam
qualities, is the only method where the response of each
individual IC is taken into account.
Material and Methods
Three waterproof IC models were selected, PTW-30013
(0.6 cm3), PTW-31010 (0.125 cm 3) and PTW-31016 (0.016
cm 3). A fourth non-waterproof IC from Nuclear Enterprise
(NE2571) was used to validate the MC process and the
different Particle Space Files (PSF) used in the MC
simulations. Three different geometries were defined for
each of the PTW IC using information about the geometric
tolerances provided by the manufacturer, which were
labelled nominal, maximum and minimum. The maximum
geometry was defined as the maximum cavity walls and
the minimum dimensions for the central electrode
together with the minimum geometry with the minimum
cavity walls and the maximum central electrode. k QQo
values were determined, for the ten geometries defined,
at three energies (TPR20,10) 6 MV (0.674), 15 MV (0.757) and
18 MV (0.778) using MC simulation performed with the
PENELOPE code system, using PenEasy as the main
program.
Results
Differences in the active collecting volume for the three
PTW ionization chambers affects the ND,w,Qo coefficients to
the same proportion, and their influence on the kQ,Qo is
less than 0.5% ±0.2% (sigma=1) (Table 1)
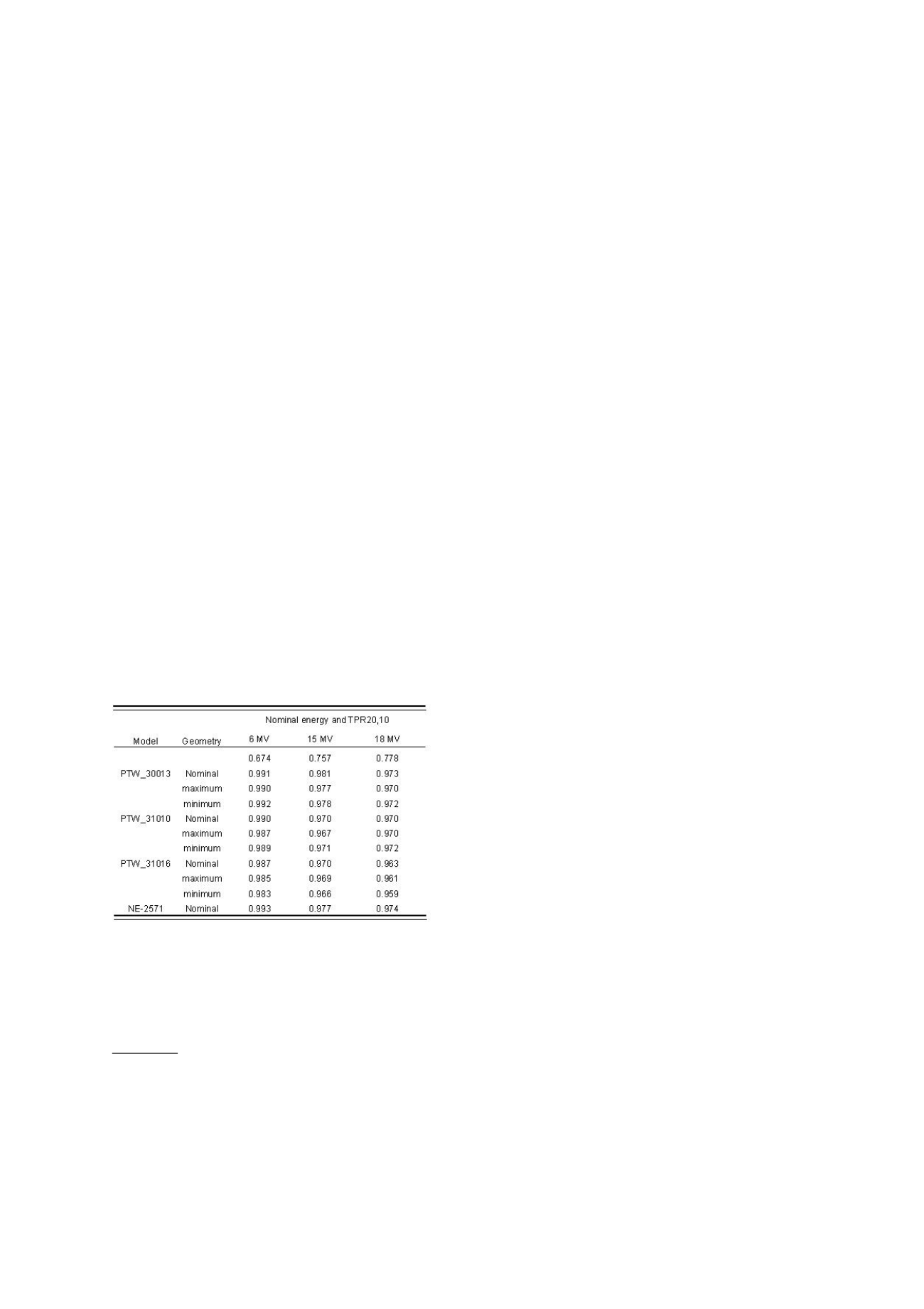
Table 1. kQQo factors determined by simulation for the
different geometries defined on each ionization chamber
at the different energies. Uncertainties on all values are
smaller than 0.2% (sigma =1).
Conclusion
The results provide an estimate of the influence that
geometrical uncertainties in the manufacturing process
have on kQQo, identifying the differences on wall thickness
as the main source of influence.
PV-0420 Learn before you measure: Method of single-
isolated errors analysis for ArcCheck.
M. Gizynska1,2, M. Bukat2, J. Cybowska2, M. Filipek2, M.
Garbacz2, I. Scisniak2, A. Spyra 2, D. Szalkowski3, A.
Walewska1
1 The Maria Sklodowska-Curie Memorial Cancer Center,
Medical Physics Department, Warsaw, Poland
2 University of Warsaw, Faculty of Physics, Department of
Biomedical Physics
3 Warsaw University of Technology, Faculty of Physics,
Warsaw, Poland
Purpose or Objective
IMRT and VMAT are nowadays techniques used in therapy
for many cancer sites. The independent, pre-treatment
verification in this type of irradiation is recommended and
in some countries – even required. There are different
ways of pre-treatment verification and there are many
phantoms and softwares design for this. One of them are
ArcCheck and SNCPatient (designed by SunNuclear).
During gamma evaluation [Depuydt, 2002] it is hard to
detect different sources of errors (both phantom and
machine). That is the reason to test a method of analysing
isolated errors and their influence on gamma results. The
method described below can show the dominant sources
of errors helping physicists in interpreting gamma
evaluation results.
Material and Methods
We divided sources of errors into two groups: phantom
based (e.g. dose rate (DR) dependence, inclinometer
tolerance) and machine based (e.g. gantry angle and leaf
positions errors). For all types of errors we prepared an
ideal dose distribution (calculated in Eclipse TPS and
imported into SNCPatient Software) and a measurement
file with an error (MWE). The diodes readouts were
calculated from TPS dose distribution. Errors were
introduced or into TPS itself (inclinometer tolerance, leaf
and gantry positions error) or outside the TPS in Python
script (DR dependence of each diode). The resolution of
readouts in the MWE were identical to those of real
measurement.
Results
The influence of ArcCheck DR dependence on VMAT plans
results is shown in Table. In about 14% of patients with the
mean DR below 100MU/min gamma did not reach 95%
passing rate. It means that in case of low DR reason of plan
failure can be caused by ArcCheck DR dependence not by
wrong realisation. The examples of leaf influence errors
are shown in Figure. It can be seen that gap width error is
more important for highly modulated plans (H&N versus
brain plans). Even some 1mm errors can be detected with
global gamma 3mm/3% method which is a good result. In
the same time it would be more complicated to detect
2mm systematic shift in leafs which can be connected with
phantom position error or misalignment of radiation field
defined by MLC. Inclinometer tolerance is the same as
gantry positioning tolerance. ArcCheck rotation error and
gantry position error can sum up. Rotation error of 0.5deg
cannot be seen in gamma 2mm/2%. In the same case dose
difference of more than 3% can be seen in high gradient
region resulting in lower DTA passing rate. Rotation error
does not seem to be symmetrical which can be connected
with diodes placement. Tilt error of 0.5deg cannot be seen
in gamma 2mm/2% and is symmetrical.